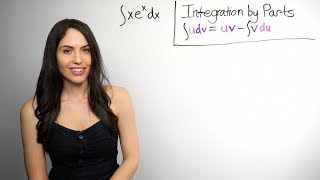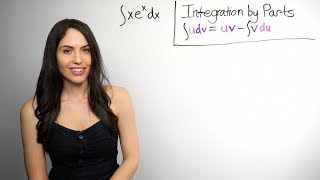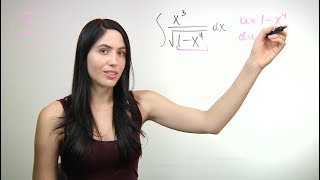
How to Integrate Using U-Substitution (NancyPi)
Channel: NancyPi
Category: Education
Tags: how tosubstitutionconstantmathematicscosderivativeexponentsrulesinside functionexplanationcalculussubstitutedifferentialexamplerearrangejmtdudifferentiatehow-tou subintegralrearrange the uoutside functionsinchain rulehow do yousquare rootusubintegration by substitutionvariablestrignancyintegratefractiondenominatornumeratorhelpu-substitutionintegrationpower rulekhannancypimathrearrange the duindefinite integralanti-derivative
Description: MIT grad shows how to do integration using u-substitution (Calculus). To skip ahead: 1) for a BASIC example where your du gives you exactly the expression you need in order to substitute, skip to time 1:30. 2) For an example where you have to REARRANGE THE DU by multiplying or dividing because the du has a different number or sign than what you need, skip to time 8:21. 3) For one where you have to REARRANGE THE U by subtracting or adding because the du expression cannot give you the expression you need, skip to 17:15. 4) For u-substitution with TRIG (SIN/COS) and the power rule, skip to 22:35. Nancy formerly of MathBFF explains the steps. Follow Nancy on Instagram: instagram.com/nancypi Twitter: twitter.com/nancypi For my video on the BASICS of INTEGRATION, jump to: youtu.be/e1nxhJQyLYI With all u-substitution integration problems: The FIRST STEP is to pick your "u". The best choice is usually the longer x-expression that is inside a power or a square root or the denominator, etc (in an "inside function"). Set u equal to this x-expression. The SECOND STEP is to find "du" by taking the derivative of the u expression with respect to x. For instance, if you have u=3x+2, your du would then be du=3dx. **NOTE: Remember to include "dx" at the end of your du differential expression. The THIRD STEP is to substitute u and du into the integral everywhere in place of x and dx. **NOTE: if your du does not perfectly match what you need in order to completely substitute before integrating, you must rearrange the du, or sometimes rearrange the u, in order to fully substitute before integrating. For an example of each, see example #2 (time: 8:21) and example #3 (time: 17:15). The FOURTH STEP is to integrate, remembering to add "+ C" at the end since you integrated an indefinite integral (no limits). The du goes away when you integrate. The LAST STEP is to "back-substitute" by replacing everywhere u appears with the x-expression that you chose u to be. For more of my math videos, check out: nancypi.com